
The lines have the same slope and different y-intercepts. A system of linear equations has either no solutions (inconsistent), one solution, or an infinite number of solutions (dependent). Thus, there are an infinite number of solutions.Īnother type of system of linear equations is an inconsistent system, which is one in which the equations represent two parallel lines. Every point on the line represents a coordinate pair that satisfies the system.

In other words, the lines coincide so the equations represent the same line. Determine all possibilities for the number of solutions of each of the system of linear equations described below.

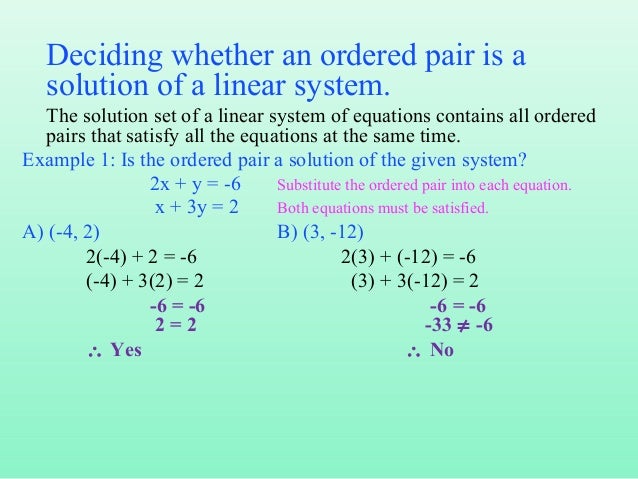
A consistent system is considered to be a dependent system if the equations have the same slope and the same y-intercepts. A system of simultaneous linear equations can have either: one unique solution, infinitely many solutions or no solutions. The two lines have different slopes and intersect at one point in the plane. A consistent system is considered to be an independent system if it has a single solution, such as the example we just explored. A solution for a system of linear Equations can be found by using the inverse of a matrix. A consistent system of equations has at least one solution. Consider the system of equations: 3 x 1 + 8 x 2 10. Linear equation has one, two or three variables but not every. Any row or linear multiple of a row can be added/subtracted to/from another row without changing the solution of the linear system. A linear equation is an algebraic equation in which the highest exponent of the variable is one. In addition to considering the number of equations and variables, we can categorize systems of linear equations by the number of solutions. Any row can be multiplied by a constant without changing the solution of the linear system. For example, consider the following system of linear equations in two variables.Ģ ( 4 ) + ( 7 ) = 15 True 3 ( 4 ) − ( 7 ) = 5 True \begin 2 ( 4 ) + ( 7 ) = 15 True 3 ( 4 ) − ( 7 ) = 5 True The three cases of systems of linear equations are: Consistent and independent (one unique solution), inconsistent (no solution) and dependent (all ordered pairs of the first equation are also ordered pairs of the other equations).Linear equations could be in the form: standard form, slop-intercept form, two point form and point-slope form. In this section, we will look at systems of linear equations in two variables, which consist of two equations that contain two different variables. Even so, this does not guarantee a unique solution. This is useful when you just need a rough answer, or youre pretty sure the intersection happens at. In order for a linear system to have a unique solution, there must be at least as many equations as there are variables. Solving Systems of Linear Equations The Graphing Method. Step 3: Determine the system’s solution by identifying the intersection point if the lines cross. Step 2: Determine whether the lines are parallel, intersect, or overlap. Step 1: Graph both equations on a rectangular plane. Some linear systems may not have a solution and others may have an infinite number of solutions. Step by step procedure to solve a system of linear equations by graphing. To find the unique solution to a system of linear equations, we must find a numerical value for each variable in the system that will satisfy all equations in the system at the same time. Solve a system of linear equations by graphingĪ system of linear equations consists of two or more linear equations made up of two or more variables such that all equations in the system are considered simultaneously.Determine whether an ordered pair is a solution to a system of linear equations.Recognize consistent and inconsistent, dependent and independent systems of linear equations.Which is the same as the matrix form given by user153582. So you're looking for a $T:\mathbbīut there are supposed to be variables on the left side in order to change 3 equations in three unknowns to matrix form.


 0 kommentar(er)
0 kommentar(er)
